Mean Value Theorem and Taylor's Theorem

Rst by subtracting a linear ie. In the proof of the Taylors theorem below we.

Section 9 7 Taylor Theorem Taylor S Theorem Like All Of The Value Theorems This Is An Existence Theorem Ppt Download

X a k R n 1 x where the error term R n 1 x satisfies R n 1 x f n 1 c n 1.

. Then for each x in the interval f x k 0 n f k a k. Applying the Mean Value Theorem for integrals on the factor. Mean Value Theorem for Vector Value Functions Theorem 519 Suppose f is.

TU The mean value theorem and Taylors expansion are powerful tools in statistics that are used to derive estimators from nonlin- ear estimating equations and to study the asymptotic properties of the resulting estimators. Using just the Mean Value Theorem we prove the nth Taylor Series Approximation. Remember that the Mean Value Theorem only gives the existence of such a point c and not a method for how to find c.

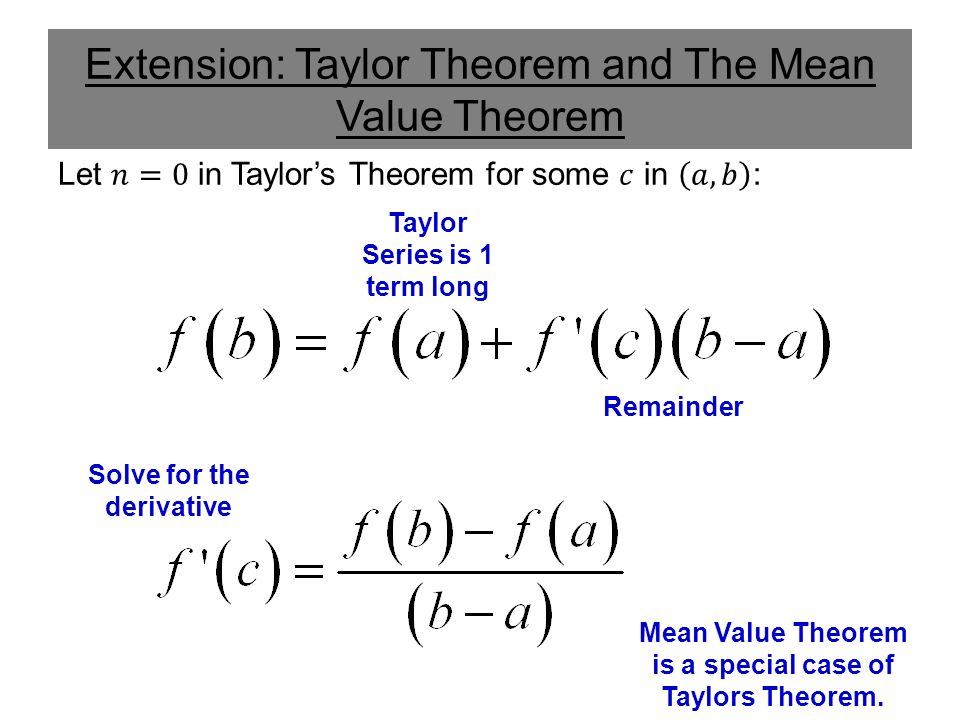
But now look again at the mean value theorem alias Taylors theorem with N 0. Degree 1 polynomial we reduce to the case where fa fb 0. Also other similar expressions can be found.

Generalizing the Mean Value Theorem Taylors theorem We explore generalizations of the Mean Value Theorem which lead to error estimates for Taylor polynomials. F x f x is continuous on the closed interval ab a b. Then there is a number c c such that a c b and.

Suppose f x f x is a function that satisfies both of the following. 1 Mean Value Theorem Let hx be differentiable on ab with continuous derivative. Mean Value Theorem.

F x he i f x hf x θ he i for some θ 0 1. Ad Find Calculus Lessons That Cover Everything Youll Be Taught in Class Plus Much More. Extended Mean Value Theorem If f and f0 are continuous on ab and f0 is difierentiable on ab then there exists c 2 ab such that fb faf0aba f00c 2 ba2.

Suppose f has n 1 continuous derivatives on an open interval containing a. We establish analogues of the mean value theorem and Taylors theorem for fractional differential operators defined using a Mittag-Leffler kernel. Here fa is a 0-th degree Taylor polynomial.

T o see this let. Then we test this generalization on polynomial functions. These refinements of Taylors theorem are usually proved using the mean value theorem whence the name.

Then for any there exists such that. Fz fracfy - fxy-x fracR_2yy-x textor R_2y fzy-x Taking anti-derivative with respect to y where fz is just a constant here we have. It says that if f is di erentiable then fxfaf0cxa and so if jxaj then jfx faj max c2ax jf0cj.

Improve your grades with step-by-step help test prep and your own study planner. The proof of the mean-value theorem comes in two parts. F x f x is differentiable on the open interval ab a b.

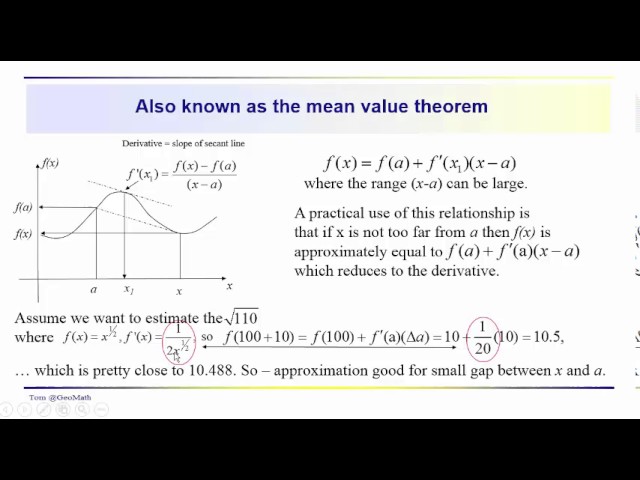
Let be a n-times differentiable function on the interval. For any in ab by Lagranges mean value theorem there exists 2. The Mean Value Theorem of Derivatives allows one to make judgments about the accuracy of such approximations and how their accuracy may improve by decreasing the distance between adjacent values of the function used for the approximations.

If f is a C 1 real v alued function on an op en set U R n w e ha v e for an y x h i with k h k sufficien tly. Use the Integral Version of Taylors theorem to write. It is a desirable property that the Taylor.

X ak plus an error that is at most max acx f n1cxa 1. Recall that the mean value theorem says that given a continuous function f on a closed interval. R_2y frac12fzy-x2 which gives the result you may be looking for.

The Mean Value Theorem and Taylors Expansion in Statistics Changyong FENG Hongyue WANGYuHAN Yinglin XIA and Xin M. Taylors Theorem Di erentiation of Vector-Valued Functions Taylors Theorem Theorem 515 Suppose f is a real function on ab n is a positive integer f n 1. Namely if f is differentiable at least n 1 times on ab then x ab fx P n k0 fka.

This result is a particular case of Taylors Theorem whose proof is given below. This can be considered to be a second-order Mean Value Theorem. Math 51H Mean v alue theorem T a ylors theorem and in tegrals.

This lemma implies the k2 case of Taylors Theorem since we have beginalign R_a2h fah - left fa h fa frac h22faright frac h22 left fatheta h - faright. Taylors Theorem Mean Value Version. The Taylor Series can also be used to approximate the value of a function at a nearby base value.

Suggests that we may modify the proof of the mean value theorem to give a proof of Taylors theorem. Next the special case where fa fb 0 follows from Rolles theorem. The important thing about this inequality is that it says that the di erence between fxandfa is at worst linear in the distance between x and a.

Mean Value Theorem and Taylors Theorem Mean value theorem LHopitals rule Taylors theorem Theorem If f x is a di erentiable function such that f 0x 0 for any x 2ab then f x is strictly increasing. Suppose f 0x 0 for any x 2ab. For example if G t is continuous on the closed interval and differentiable with a non-vanishing derivative on the open interval between a and x then for some number ξ between a and x.

We understand this equation as saying that the difference between fb and fa is given by an expression resembling the next term in the Taylor polynomial. We formulate a new model for the fractional Boussinesq equation by using this new Taylor series expansion. So by Mean Value Theorem exists z in x y st.

If we take b x and a x0 in the previous result we obtain that. Formulae 6 and 10 obtained for Taylors theorem in the ABC context appear different from classical and previous results mainly due to the replacement of power functions with a more general form of summand. In this manuscript we have proved the mean value theorem and Taylors theorem for derivatives defined in terms of a MittagLeffler kernel.

Calculus Mean Value Theorem And The Taylor Series Youtube

Calculus Continuity Of F N 1 In Taylor S Theorem With Mean Value Remainder Mathematics Stack Exchange

How Is Taylor S Theorem Like The Mean Value Theorem Week 6 Lecture 7 Sequences And Series Youtube